Degrees, Turnings, and "Bumps"
Purplemath
Graphs of polynomials don't always head in just one direction, like nice neat straight lines. Instead, they can (and usually do) turn around and head back the other way, possibly multiple times. Each time the graph goes down and hooks back up, or goes up and then hooks back down, this is a "turning" of the graph.
I refer to the "turnings" of a polynomial graph as its "bumps". This isn't standard terminology, and you'll learn the proper terms (such as "local maximum" and "global extrema") when you get to calculus, but, for now, we'll talk about graphs, their degrees, and their "bumps".
For instance, the following graph has three bumps, as indicated by the arrows:
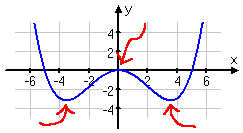
Content Continues Below
Below are graphs, grouped according to degree, showing the different sorts of "bump" collection each degree value, from two to six, can have. Compare the numbers of bumps in the graphs below to the degrees of their polynomials. In particular, note the maximum number of "bumps" for each graph, as compared to the degree of the polynomial:
degree two:
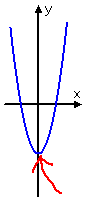
one bump
degree three:
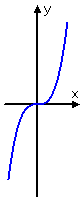
zero bumps, but one flex point
degree three:

two bumps
degree four:
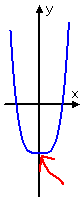
one (flattened) bump
degree four:
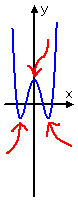
three bumps
degree five:

zero bumps, but one flex point
degree five:
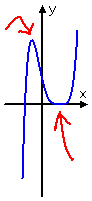
two bumps (one flattened)
degree five:
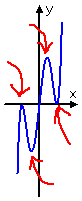
four bumps
degree six:
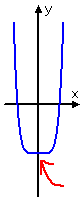
one (flat) bump
degree six:
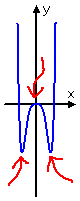
three bumps (one flat)
degree six:
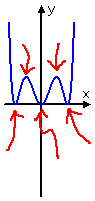
five bumps
You can see from these graphs that, for degree n, the graph will have, at most, n − 1 bumps. The bumps represent the spots where the graph turns back on itself and heads back the way it came. This change of direction often happens because of the polynomial's zeroes or factors. But extra pairs of factors (from the Quadratic Formula) don't show up in the graph as anything much more visible than just a little extra flexing or flattening in the graph.
Affiliate
Because pairs of factors have this habit of disappearing from the graph (or hiding in the picture as a little bit of extra flexture or flattening), the graph may have two fewer, or four fewer, or six fewer, etc, bumps than you might otherwise expect, or it may have flex points instead of some of the bumps. That is, the degree of the polynomial gives you the upper limit (the ceiling) on the number of bumps possible for the graph (this upper limit being one less than the degree of the polynomial), and the number of bumps gives you the lower limit (the floor) on degree of the polynomial (this lower limit being one more than the number of bumps).
We can use this information to make some intelligent guesses about polynomials from their graphs, and about graphs from their polynomials. For instance:
-
What is the minimum possible degree of the polynomial graphed below?
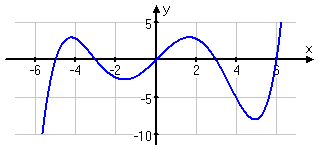
Given a polynomial's graph, I can count the bumps. The degree of the polynomial will be no less than one more than the number of bumps, but the degree might be three more than that number of bumps, or five more, or....
Since there are four bumps on the graph, and since the end-behavior confirms that this is an odd-degree polynomial, then the degree of the polynomial is 5, or maybe 7, or possibly 9, or... But this exercise is asking me for the minimum possible degree. So my answer is:
The minimum possible degree is 5.
Content Continues Below
-
Given that a polynomial is of degree six, which of the following could be its graph?


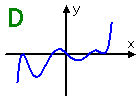
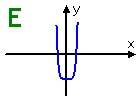
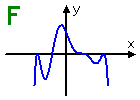


To answer this question, I have to remember that the polynomial's degree gives me the ceiling on the number of bumps. In this case, the degree is 6, so the highest number of bumps the graph could have would be 6 − 1 = 5. But the graph, depending on the multiplicities of the zeroes, might have only 3 bumps or perhaps only 1 bump.
(I would add 1 or 3 or 5, etc, if I were going from the number of displayed bumps on the graph to the possible degree of the polynomial, but here I'm going from the known degree of the polynomial to the possible graph, so I subtract. ). If you're not sure how to keep track of the relationship, think about the simplest curvy line you've graphed, being the parabola. It has degree two, and has one bump, being its vertex.)
Also, I'll want to check the zeroes (and their multiplicities) to see if they give me any additional information. I'll consider each graph, in turn.

Graph A: This shows one bump (so not too many), but only two zeroes, each looking like a multiplicity-1 zero. This is probably just a quadratic, but it might possibly be a sixth-degree polynomial (with four of the zeroes being complex). I would have expected at least one of the zeroes to be repeated, thus showing flattening as the graph flexes through the axis. But this could maybe be a sixth-degree polynomial's graph.

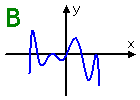
Graph B: This has seven bumps, so this is a polynomial of degree at least 8, which is too high. This graph cannot possibly be of a degree-six polynomial.

Graph C: This has three bumps (so not too many), it's an even-degree polynomial (being "up" on both ends), and the zero in the middle is an even-multiplicity zero. Also, the bump in the middle looks flattened at the axis, so this is probably a repeated zero of multiplicity 4 or more. With the two other zeroes looking like multiplicity-1 zeroes, this is very likely a graph of a sixth-degree polynomial.

Graph D: This has six bumps, which is too many; this is from a polynomial of at least degree seven. On top of that, this is an odd-degree graph, since the ends head off in opposite directions. So this can't possibly be a sixth-degree polynomial.

Graph E: From the end-behavior, I can tell that this graph is from an even-degree polynomial. The one bump is fairly flat, so this is more than just a quadratic. This might be the graph of a sixth-degree polynomial.

Graph F: This is an even-degree polynomial, and it has five bumps (and a flex point at that third zero). But looking at the zeroes, the left-most zero is of even multiplicity; the next zero passes right through the horizontal axis, so it's probably of multiplicity 1; the next zero (to the right of the vertical axis) flexes as it passes through the horizontal axis, so it's of multiplicity 3 or more; and the zero at the far right is another even-multiplicity zero (of multiplicity two or four or...). Adding these up, the number of zeroes is at least 2 + 1 + 3 + 2 = 8 zeroes, which is way too many for a degree-six polynomial. The bumps were right, but the zeroes were wrong. This can't possibly be a degree-six graph.

Graph G: The graph's left-hand end enters the graph from above, and the right-hand end leaves the graph going down. Since the ends head off in opposite directions, then this is another odd-degree graph. As such, it cannot possibly be the graph of an even-degree polynomial, of degree six or any other even number.

Graph H: From the ends, I can see that this is an even-degree graph, and there aren't too many bumps, seeing as there's only the one. Looking at the two zeroes, they both look like at least multiplicity-3 zeroes. So this could very well be a degree-six polynomial.
So I've determined that Graphs B, D, F, and G can't possibly be graphs of degree-six polynomials. Graphs A and E might be degree-six, and Graphs C and H probably are. This question asks me to say which of the graphs could represent the graph of a polynomial function of degree six, so my answer is:
Graphs A, C, E, and H
Affiliate
Affiliate
To help you keep straight when to add and when to subtract, remember your graphs of quadratics and cubics. Quadratics are degree-two polynomials and have one bump (always); cubics are degree-three polynomials and have two bumps or none (having a flex point instead). So going from your polynomial to your graph, you subtract, and going from your graph to your polynomial, you add. If you know your quadratics and cubics very well, and if you remember that you're dealing with families of polynomials and their family characteristics, you shouldn't have any trouble with this sort of exercise.
URL: https://www.purplemath.com/modules/polyends4.htm
Select a Course Below
Standardized Test Prep
Homeschool Math
© 2024 Purplemath, Inc. All right reserved. Web Design by ![]()



