Return to the Purplemath home page

 

Return to the Lessons Index  | Do the Lessons in Order  |  Print-friendly page

Graphing Logarithmic Functions: Examples (page 2 of 3)

In the previous example, I said there were two options for how to graph logs. The previous page demonstrated how to work from the concept of logarithms to find nice neat points to plot. However, the other option is that you can use your calculator to find plot points.

  • Graph y = log2(x)
     

    To find my plot-points using my calculator (since my calculator can only compute common, or base-10, and natural, or base-e, logs), I will need to use the change-of-base formula, which gives me an equivalent equation. The original equation, y = log2(x) becomes y = ln(x)/ln(2):

      

    y1 = ln(x) / ln(2)
      

      

    Once I've entered the calculator-friendly form of the equation, I can get some plot points from my calculator's TABLE feature:

      Copyright © Elizabeth Stapel 2002-2011 All Rights Reserved

    (If you have an old TI-85, so you have no in-built "TABLE" utility, you can install any of the various after-market programs which do much the same thing. The graphics to the right are screen shots of what the program on my TI-85 produced.)

    I know I have to have all positive -values inside the log, so I start the TABLE listing at x = 1, and go from there.

      

    TABLE for x = 1 to x = 6

    TABLE for x = 7 to x = 12

    TABLE for x = 13 to x = 18
      

    I'd still like to have some plot points between zero and one, so I adjust the initial ("start") value and the increment (the "count by" amount) to get some additional plot points between x = 0 and x = 1:

     

      

    TABLE for x = 1/8 to x = 7/8, counting by 1/8's

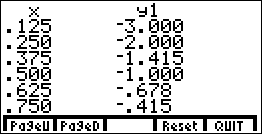
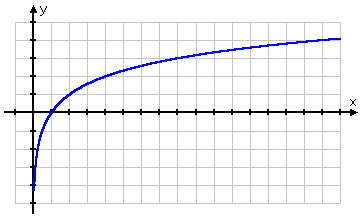
    Using these points (plotted to one or two decimal places in "accuracy"), I will end up with the same graph as before.

     

    graph of y = log_2(x)

Depending upon your calculator's software, you will either get blank spaces in your TABLE for the y-values when x = 0 and when x is negative; or the slot will display "ERROR", "UNDEFINED", or some other error code; or else the program will crash. (Mine crashes for undefined y-values, which is why I was careful to start my TABLE display above at a positive x-value.) This behavior in the TABLE feature reinforces the fact that logarithms are not defined for non-positive arguments.

(Regarding finding plot points between x = 0 and x = 1, if you do not know how to change your initial value from x = 0 or how to change your increment from 1, consult your owner's manual; the instructions will be somewhere in the chapter on graphing.)

If you are graphing the common (base-10) log or the natural (base-e) log, just use your calculator to get the plot points. When working with the common log, you will quickly reach awkwardly large numbers if you try to plot only whole-number points; for instance, in order to get as high as y = 2, you'd have to use x = 100, and your graph would be ridiculously wide. When working with the natural log, the base e is an irrational number anyway, so there's no point in even trying to find nice neat plot points, because, other than (1, 0), there aren't any.

Sometimes the log graph is shifted a bit from the "usual" location (shown in the graph above), either up, down, right, left, or upside-down, or else some combination of these. But the general shape of the graph tends to remain the same.

  • Graph y = log3(x) + 2.

    This is the basic log graph, but it's been shifted upward by two units. To find plot points for this graph, I will plug in useful values of x (being powers of 3, because of the base of the log) and then I'll simplify for the corresponding values of y.

      30 = 1, so log3(1) = 0, and log3(1) + 2 = 2
      31 = 3,       so log3(3) = 1, and log3(3) + 2 = 3
      32 = 9,       so log3(9) = 2, and log3(9) + 2 = 4
      33 = 27,       so log3(27) = 3, and log3(27) + 2 = 5

    Moving in the other direction (to get some y-values for x between 0 and 1):

      3–1 = 1/3, so log3( 1/3 ) = –1, and log3( 1/3 ) + 2 = 1
      3–2 = 1/9,       so log3( 1/9 ) = –2, and log3( 1/9 ) + 2 = 0
      3–3 = 1/27,       so log3( 1/27 ) = –3, and log3( 1/27 ) + 2 = –1

    These are the only "neat" points that I'm going to bother finding for my graph. If I feel a need for additional plot points, especially between any two of the points I found above, I can evaluate the function "ln(x) / ln(3)" in my calculator.

      

    The graph of y = log3(x) + 2 looks like this:

      

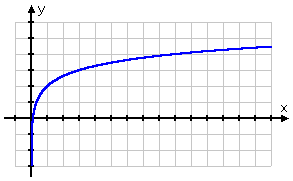
    graph of y = log_3(x) + 2 
     

<< Previous  Top  |  1 | 2 | 3  |  Return to Index  Next >>

Cite this article as:

Stapel, Elizabeth. "Graphing Logarithmic Functions: Examples." Purplemath. Available from
    https://www.purplemath.com/modules/graphlog2.htm. Accessed  
 

 

Purplemath:
  Linking to this site
  Printing pages
  School licensing

Reviews of
Internet Sites:
   Free Help
   Practice
   Et Cetera

The "Homework
   Guidelines"

Study Skills Survey

Tutoring from Purplemath
Find a local math tutor

  Copyright © 2002-2012  Elizabeth Stapel   |   About   |   Terms of Use

 

Contact Us