The Four Quadrants
Purplemath
What are the four quadrants?
The Cartesian plane has an horizontal and a vertical axis; these two axes divide the plane into four sections. These sections are called "quadrants", and are labelled with Roman numerals (not Arabic numerals), starting at the positive x-axis and going around anti-clockwise.
Content Continues Below
If we animate the movement from Quadrant I, starting from the top right section and rotating anti-clockwise through Quadrant II (abbreviated "QII") and Quadrant III ("QIII") to the bottom right section (being Quadrant IV, or QIV), we get the following:
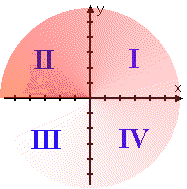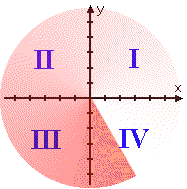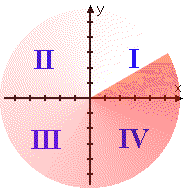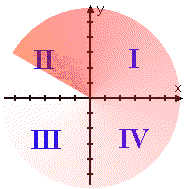
(The image above is animated on the "live" web page.)
Affiliate
("What is the English for 'anti-clockwise'?", you ask? That *is* the English; the American is "counter-clockwise".)
When you get to trigonometry, this order of numbering the quadrants will make perfect sense and will be very useful. For now, just memorize the information.
You'll probably have only a few test questions on quadrants, and then you'll hardly see the topic again until trigonometry.
Content Continues Below
- In which quadrant is the point (−2, −3)?
The simplest way for me to answer this is to plot the point:
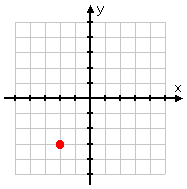
Now I can see that the point (−2, −3) is in Quadrant III.
- In which quadrant is the point (4, y)?
Since they don't tell me what the value of y is, then y can be anything. This means that (4, y) is not just one point, but is instead infinitely-many points. Here's how:
Since y can be −5, then (4, −5) is a valid answer. So is (4, −3), (4, 0), (4, 2), and (4, 4). So is any point that has first coordinate x = 4:
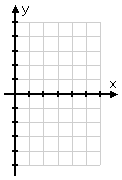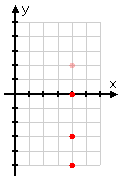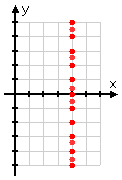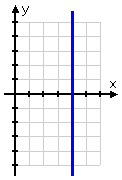
By plotting a bunch of points that work (that is, a bunch of points that fulfill the given rule), I can see that the "point" (4, y) is actually an entire line: the line "x = 4". And this line passes through two quadrants. So my answer is:
Quadrants I and IV
By the way, please use standard notation for the quadrants. You almost certainly can abbreviate "Quadrant" as just "Q", but don't completely omit the quadrant label.
And be careful to use the Roman "I, II, III, IV" for numbering the quadrants, not the Arabic "1, 2, 3, 4". If you need to denote two or more quadrants, then use "&" or "and" to join them, not "plus" or "+".
- In which quadrant is/are the point(s) (x, y) such that xy < 0?
This is asking me for a description of the points (x, y), where the coordinate x and y have values such that, when I multiply x and y together, I will get a negative number. To figure this one out, it's probably simplest if I just pick a sample point from each quadrant and see what I get.
Affiliate
Advertisement
In Quadrant I, I'll pick, say, (2, 3). The product of the coordinates is 2 × 3 = 6, which is positive (that is, greater than zero). Since any coordinate product in Quadrant I will be similarly positive (being a product of a positive x value and a positive y value), then Quadrant I is not part of my answer.
In Quadrant II, I'll pick, say, (−4, 5). The product of the coordinates is (−4) × 5 = −20, which is negative. Any other coordinate product in Quadrant II will also be negative (being the product of a negative x value and a positive y value), so Quadrant II is part of my answer.
In Quadrant III, I'll pick, say, (−2, −1). The product is (−2) × (−1) = 2, which is positive. Any other product in Quadrant III will be positive also (being the product of a negative x value and a negative y value), so Quadrant III is not part of my answer.
In Quadrant IV, I'll pick, say, (3, −4). The product is 3 × (−4) = −12, which is negative. Any other product in Quadrant IV will be negative also (being the product of a positive x value and a negative y value), so Quadrant IV is part of my answer.
I've found the two quadrants which obey the given condition; namely, the two quadrants that contain points (x, y), with the coordinates' product xy < 0 (that is, with the product being negative). My hand-in answer is:
Quadrant II and Quadrant IV
URL: https://www.purplemath.com/modules/plane3.htm
You can use the Mathway widget below to practice figuring out the quadrant in which a given point is located. Try the entered exercise, or type in your own exercise. Then click the button and select "Find the Quadrant" to compare your answer to Mathway's.
Please accept "preferences" cookies in order to enable this widget.
(Click "Tap to view steps" to be taken directly to the Mathway site for a paid upgrade.)
Select a Course Below
Standardized Test Prep
Homeschool Math
© 2024 Purplemath, Inc. All right reserved. Web Design by ![]()